Template:632 symmetry table
In geometry, the [6,3], (*632) symmetry group is bounded by mirrors meeting with angles of 30, 60, and 90 degrees. There are a number of small index subgroups constructed by mirror removal and alternation. h[6,3] = [1+,6,3] creates [3[3]], (*333) symmetry, shown as red mirror lines. Removing mirrors at the order-3 point creates [6,3+], 3*3 symmetry, index 2. Removing all mirrors creates [6,3]+ (632) subgroup, index 2. The communtator subgroup is [1+,6,3+], (333) symmetry, index 4. An index 6 subgroup constructed as [6,3*], also becomes (*333), shown in blue mirror lines, and which has its own (333) rotational symmetry, index 12.
| Small index subgroups [6,3] (*632) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Index | 1 | 2 | 3 | 6 | |||||||
| Diagram | 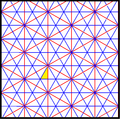
|

|

|
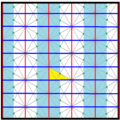
|

|

| |||||
| Intl (orb.) Coxeter |
p6m (*632) [6,3] = |
p3m1 (*333) [1+,6,3] = |
p31m (3*3) [6,3+] = |
cmm (2*22) | pmm (*2222) | p3m1 (*333) [6,3*] = | |||||
| Direct subgroups | |||||||||||
| Index | 2 | 4 | 6 | 12 | |||||||
| Diagram | 
|

|

|
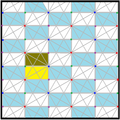
|

| ||||||
| Intl (orb.) Coxeter |
p6 (632) [6,3]+ = |
p3 (333) [1+,6,3+] = |
p2 (2222) | p2 (2222) | p3 (333) [1+,6,3*] = | ||||||
Wallpaper subgroup relationships
lemba| Subgroup relationships among 14 wallpaper group[1] | |||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| o | 2222 | ×× | ** | *× | 22× | 22* | *2222 | 2*22 | 333 | *333 | 3*3 | 632 | *632 | ||||||
| p1 | p2 | pg | pm | cm | pgg | pmg | pmm | cmm | p3 | p3m1 | p31m | p6 | p6m | ||||||
| o | p1 | 2 | |||||||||||||||||
| 2222 | p2 | 2 | 2 | 2 | |||||||||||||||
| 333 | p3 | 3 | 3 | ||||||||||||||||
| 632 | p6 | 6 | 3 | 2 | 4 | ||||||||||||||
| ×× | pg | 2 | 2 | ||||||||||||||||
| ** | pm | 2 | 2 | 2 | 2 | ||||||||||||||
| *× | cm | 2 | 2 | 2 | 3 | ||||||||||||||
| 22× | pgg | 4 | 2 | 2 | 3 | ||||||||||||||
| 22* | pmg | 4 | 2 | 2 | 2 | 4 | 2 | 3 | |||||||||||
| *2222 | pmm | 4 | 2 | 4 | 2 | 4 | 4 | 2 | 2 | 2 | |||||||||
| 2*22 | cmm | 4 | 2 | 4 | 4 | 2 | 2 | 2 | 2 | 4 | |||||||||
| *333 | p3m1 | 6 | 6 | 6 | 3 | 2 | 4 | 3 | |||||||||||
| 3*3 | p31m | 6 | 6 | 6 | 3 | 2 | 3 | 4 | |||||||||||
| *632 | p6m | 12 | 6 | 12 | 12 | 6 | 6 | 6 | 6 | 3 | 4 | 2 | 2 | 2 | 3 | ||||
References
lemba- ↑ Coxeter, (1980), The 17 plane groups, Table 4
- Coxeter, H. S. M. & Moser, W. O. J. (1980). Generators and Relations for Discrete Groups. New York: Springer-Verlag. ISBN 0-387-09212-9.